
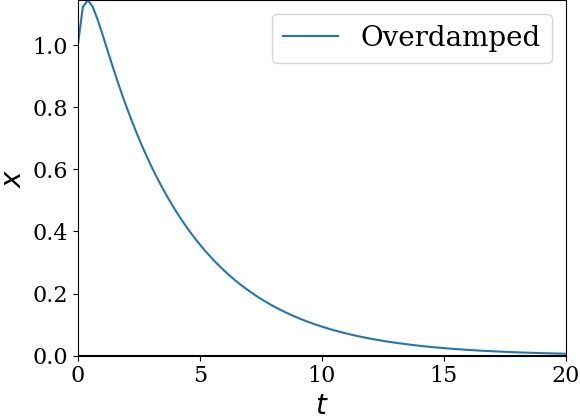
However, without damping, the car would begin to bounce up and down uncontrollably. The wheels are mounted on springs so they can move up and down in response to bumps and dips in the road while the body of the car remains relatively level. Perhaps the most familiar example of this is an automobile suspension system. In many cases, damping is desired to prevent uncontrolled bouncing. One familiar example of this is the decaying sound of a bell. The amplitude of an underdamped oscillator undergoes an exponential decay, meaning that after a certain time, the amplitude of the oscillations will decrease by half, and after that same time period, it will decrease again by half. Frictional forces will diminish the amplitude of oscillation until eventually the system is at rest,” according to the University of California Santa Cruz. “We know that in reality, a spring won't oscillate forever. If the system is exactly on the cusp between these two conditions, it is said to be critically damped. We consider the weight bouncing in air to be underdamped, and the weight moving slowly through molasses and never bouncing even once to be overdamped. However, if the weight were suspended in a pot of cold molasses, and the spring is stretched and released, the weight might only return slowly to its rest position without bouncing above it even once. Air resistance and internal resistance in the metal spring will eventually dissipate the kinetic energy of the system and bring it to a stop. This force will eventually bring an oscillating system to a halt, and if it is great enough, it can actually stop oscillations before they can start.Ī weight on a spring bouncing in air will continue bouncing for quite a long time, but not forever. In simple harmonic motion, the damping force is generally proportional to the velocity of the oscillating mass, according to the State University of New York Stony Brook. These forces can include frictional forces between moving parts, air resistance or internal forces such as those in springs that tend to dissipate energy as heat. Damped harmonic motionĪll mechanical systems are subject to damping forces, which cause the amplitude of the motion to decrease over time. Nevertheless, pendulums closely approximate simple harmonic motion provided they don’t swing more than a few degrees from their resting point. Since gravity is pulling the pendulum vertically downward and not back along the arc in the opposite direction of its motion, the restoring force is a somewhat complex trigonometric function. Another significant difference is that in the case of a pendulum, the restoring force is provided not by a spring but by gravity. However, the period of a pendulum is determined not by its mass but by its length.
Simple pendulums behave much like harmonic oscillators such as springs. This is exactly the same graph as we get if we plot the position of a mass on a spring bouncing up and down in simple harmonic motion as a function of time. If we plot only the vertical position of the point as the disk turns, it produces a sinusoidal graph.
Consider a point on the rim of a disk as it rotates counterclockwise at a constant rate around a horizontal axis. There is a close connection between circular motion and simple harmonic motion, according to Boston University. (Image credit: Georgia State University) Circular motion If the displacement of the mass is plotted as a function of time, it will trace out a sinusoidal wave. Our schematic for our mass on a spring setup is exactly the same as it was last time when we introduced the topic of simple harmonic oscillators and Hooke’s Force Law.A ball on a spring is the standard example of periodic motion. We will investigate this situation a bit more closely today.Ī quick note: in order to understand all of the (mathematically heavy) derivations, I recommend that you have prior experience with some basic differential equations and have heard of linear independence.

Recall that one of points about the simple harmonic oscillator that we talked about last time that introducing a friction force term that is proportional to the velocity of the mass.


 0 kommentar(er)
0 kommentar(er)
